Capacitors: Using Charge Sensors, Lab Report Example

Objectives

The aim of this experiment is to understand the basics of parallel plate conductors and how distance affects capacitance of a parallel plate conductors.

Procedure

Part I

We used a power supply to charge a capacitor; then carefully touched that capacitor’s leads to the leads of a second capacitor (uncharged in most cases) so that the charge on the first capacitor is shared with the second.

We mounted a 0.1 ?F capacitor on the Styrofoam block. We used the power supply to charge this capacitor to a voltage of 5.0 V; then disconnected the power supply. We attempted to measure the capacitor voltage directly with a digital voltmeter (DVM). We observed the voltage on the DVM dropping rather quickly to zero.

We then mounted the 0.1 ?F capacitor leads to the input of the charge sensor. Again we used the power supply to charge this capacitor to a voltage of 5.0 V; this time the reading should showed a constant 5.0 V on the computer display. We discharged the capacitor by touching both capacitor leads simultaneously with an alligator clip. Once again the voltage noticeably dropped rather quickly to zero. We also observed some short lived “rebound” in the voltage due to a lingering polarization in the dielectric of the capacitor.

For the formal experiment, we began with a pair of capacitors in parallel. We left the 0.1 ?F capacitor mounted on the charge sensor and mount a 1.0 ?F capacitor on the Styrofoam block; we connected the two capacitors (the connectors with small hooks are useful here) to create 1.1 ?F capacitor. We made sure the 1.1 ?F is completely discharged. We then charged a second 1.0 ?F capacitor to 5.0 V (we did this by holding the second capacitor by its plastic cover and inserted its leads into the power-supply outlets) and touch it to the uncharged 1.1 ?F capacitor (we were sure to connect positive to positive). For safety, we discharged the “charge-transfer” capacitor before putting it down. We then compared the measured voltage to what is expected from a theoretical calculation.

We then discharged the 1.1 ?F capacitor and disconnected the 1.0 ?F capacitor from the Styrofoam block. In its place, we connected a 0.1 ?F capacitor. We made sure the 0.1 ?F capacitor that is left is completely discharged we repeated the above steps using this capacitor and compared the reading to what is expected form a theoretical calculation.

This time, we did not discharge the 1.1 ?F capacitor. We recharged the 0.1 ?F charge – transfer capacitor to 5.0 V and connected it again across the 1.1 ?F capacitor, taking care to maintain the proper polarity. We observed that the increase in the voltage is a little less this time. We compared the voltage reading to what is expected from a theoretical calculation and gave an appropriate explanation as to why the increase in voltage is a little less for the second charge transfer.

We then charged the 1.1 ?F capacitor to 5.0 V. We shared the charge on this capacitor with an uncharged capacitor of unknown capacitance (the capacitor covered with black electrical tape), and from the final reading of the voltage we determine the unknown capacitance. We shared the charge by holding the uncharged capacitor and touching its leads to the leads of the charged 1.1 ?F capacitor. We reported this measurement of capacitance and compared with a second measurement taken directly with the capacitance capability of the DVM.

Part II

Finally, we wished to measure the capacitances of a length of coaxial cable and of a parallel-plate capacitor. We constructed the parallel-plate capacitor by inserting the waxed paper between the aluminium plates; pressed the combination together by putting a 1.0 kg weight on top of the top plate. Both of these capacitances were quite small, so we needed to alter our standard capacitor. We removed the 0.1 ?F capacitor and mounted a 0.01 ?F capacitor (the smaller brown capacitor: check with capacitance capability of the DVM) on the charge sensor; we disconnected the capacitor mounted on the Styrofoam. We took into account the small capacitance of the charge sensor, which we have thus far ignored. We used the capacitance capability of the DVM to measure the combined capacitance of the 0.01 ?F capacitor and the charge sensor (it was approximately twice the capacitance of the 0.01 ?F capacitor alone); this is our standard capacitor. We charge the standard capacitor to 5.0 V using the power supply. We used the charge-sharing method (as summarized below) to determine the two unknown capacitances: capacitances of a length of coaxial cable and of a parallel- plate capacitor.

1. We charged the standard capacitor to V1 = 5.0 V. (Fig. 1).
2. We disconnected one wire between power supply and the charge sensor.
3. With a quick touch, we shared the charge of the standard capacitor and a parallel-plate capacitor (Fig. 2).
4. We recorded the resultant voltage V2 across capacitors in parallel: the standard capacitor and parallel plate capacitor.
5. We calculated the capacitance of the parallel-plate capacitor.

Experimental Data

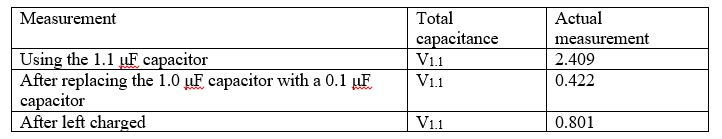
Results

Part I

From the 1mF capacitor

Q = C · V

V = Q / C

V₀ = 5V

V_1.1 = (5?1× ?10?^ (-6))/ (1.2×?10?^ (-6)) =

=2.38

Comparing this to the actual measurement

V_{1.1 actual} = 2.409 and V_{1.1 theoretical} = 2.38

Q = C · V

V = Q / C

V₀ = 5V

V_1.1 = (5 • 1×?10?^(-6))/(1.2×?10?^(-6) ) + 2.38

= 0.417 + 2.38 = 2.797

Comparing this to the actual measurement

V_{1.1 actual} = 2.831 and V_{1.1 theoretical} = 2.797

After the 1mF is left charged

Q = C · V

V = Q / C

V₀ = 5V

V_1.1 = (5 ? 1×?10?^ (-6))/ (1.2 ×?10?^ (-6)) + 2.38

= 0.799 + 2.38 = 3.179

Comparing this to the actual measurement

V_{1.1 actual} = 3.210 and V_{1.1 theoretical} = 3.179

If the 1 mF capacitor is left charged a third time

Q = C · V

V = Q / C

V₀ = 5V

V_1.1 = (5×1×?10?^ (-6))/ (1.2×?10?^ (-6)) + 2.38

= 2.599

Part II

C_{o.1 ?}F = 10 · 10^-9

C_T = 19.45 · 10^-9

V_1Kg = 1.605V

V_2Kg = 1.323V

C_1Kg =

= 3.974 x 10^-8 F

= 39.74 _?F

C_2Kg =

= 5.236 x 10^-8 F

= 52.36 _?F

For the Coaxial Cable

Q = C · V

C = Q / V

V₀ = 5V

C_coax =

= 22.217 x 10^-9 F

= 22.217 _?F

Discussion and Analysis

Part I

From the measurements of this section:

It is noted that all the actual measurements are slightly larger than the expected measurements that are derived from the mathematical application of the formula

Q = C · V

V = Q / C

This is due to the allowance for the propagation of error that is associated with the experiment. This can be due to: impurities within the given materials and/or error associated with human aspects.

It was observed that when the capacitor was left charged, the measurements depicted a much higher result. This is because if not discharged, the charge is stored and thus the stored charged if factored into the subsequent measurements.

When the capacitor was not discharged for the second time, the resultant measurement was much higher than the previous measurement.

Part II

When the two parallel plates are pressed together, their capacitances are seen to increase.

Conclusion

The capacitance of various conductor dielectric geometries is purely dependent on the distance and make-up of the material of the dielectric geometries.

There are multiple sources of error that originate from:

• Impurities within the given elements
• Human error in making measurements

The most important error is the human error as the impurity levels of the given materials cannot be determined.

The best way to reduce the error is by taking numerous measurements and using their averages.